Solution Steps P ( x ) = ( x 4 ) ( x 2 ) ( x 1 ) P ( x) = ( x 4) ( x 2) ( x − 1) Apply the distributive property by multiplying each term of x4 by each term of x2 Apply the distributive property by multiplying each term of x 4 by each term of x 2Get stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us! Click here 👆 to get an answer to your question ️ Find p(x 1) if p(x) = x^2 – 3x – 1 everkayy everkayy Mathematics High School answered Find p(x 1) if p(x) = x^2 – 3x – 1 1 See answer everkayy is waiting for your help Add your answer and earn points

If X 1 2 Is The Zero Of The Polynomial P X 8x3 Ax2 X 2 Find The Value Of A Maths Meritnation Com
P(x)=x^3-2x^2-8x-1 g(x)=x+1
P(x)=x^3-2x^2-8x-1 g(x)=x+1- The underlying distribution makes no difference Therefore, the answer is 1 / 6 Had these not been IID then you'd need to do more work and the answer would depend on how they are distributed, but in this case, the variables are exchangeable Share answered Oct 30 ' atP(X =0) = 1 16,P(X =1) = 4 16,P(X =2) = 6 16,P(X =3) = 4 16,P(X =4) = 1 16 (1) Notice that the denominators of the five fractions are the same and the numerators of the five fractions are 1, 4, 6, 4, 1 The numbers in the numeratorsis a setof binomial coefficients 1 16 = 4 0 1 16, 4 16 = 4 1 1 16, 6 16 = 4 2 1 16, 4 16 = 4 3 1 16, 1 16 = 4
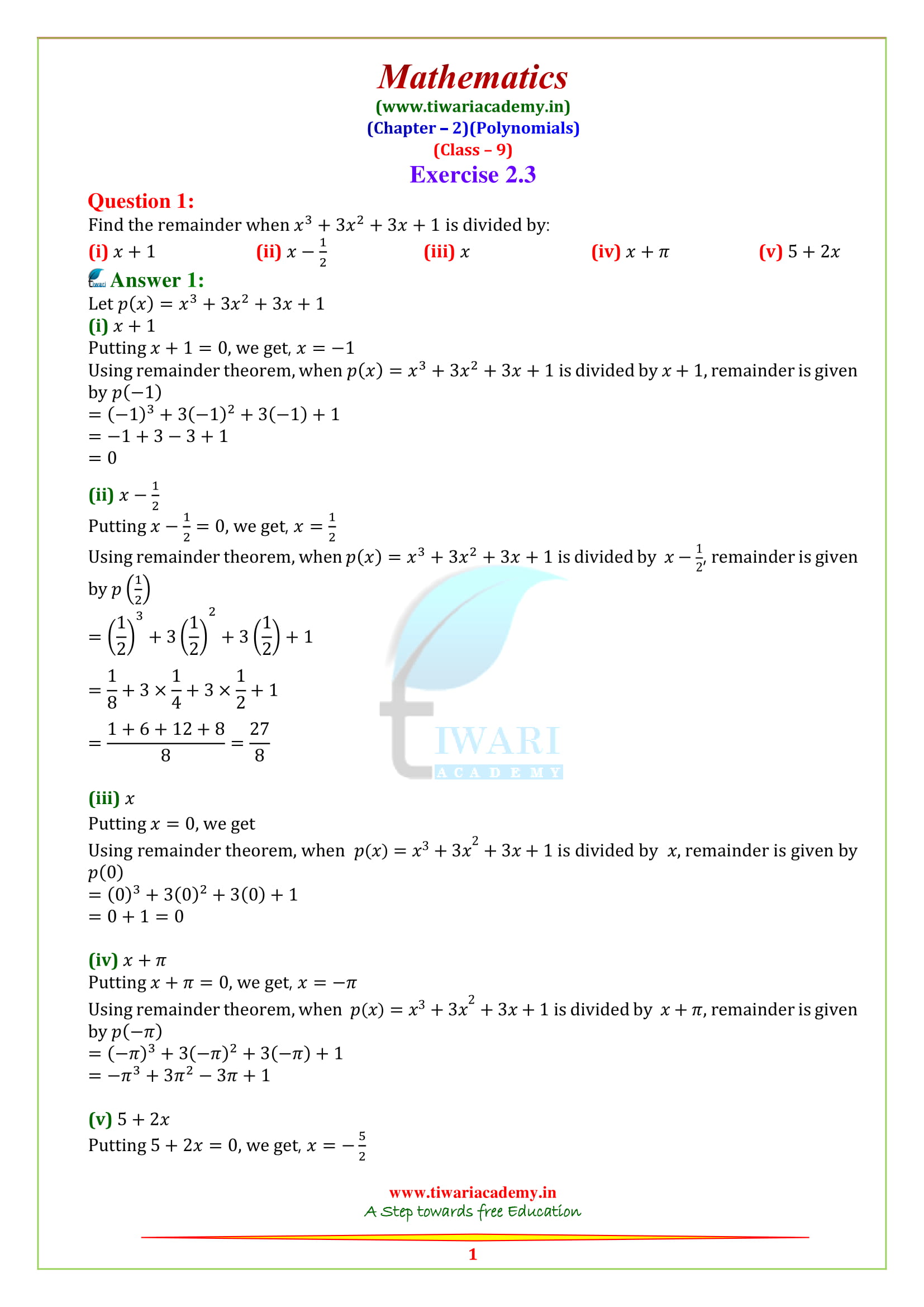



Ncert Solutions For Class 9 Maths Chapter 2 Polynomials In Pdf
1x2at Sportwetten und Casino Brasilien Campeonato Brasileiro Serie , Frauen, Playoffs 1,50The points (x,y,z) of the sphere x 2 y 2 z 2 = 1, satisfying the condition x = 05, are a circle y 2 z 2 = 075 of radius on the plane x = 05 The inequality y ≤ 075 holds on an arc The length of the arc is 5/6 of the length of the circle, which is why the conditional probability is equal to 5/6P(q(x)) p ( q ( x)) Evaluate p(q(x)) p ( q ( x)) by substituting in the value of q q into p p p(2x−1) = −2(2x−1) 1 p ( 2 x 1) = 2 ( 2 x 1) 1 Simplify each term Tap for more steps Apply the distributive property p ( 2 x − 1) = − 2 ( 2 x) − 2 ⋅ − 1 1 p ( 2 x 1) = 2 ( 2 x) 2 ⋅ 1 1 Multiply 2 2 by −
Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historyThe probability of having x successes in n trials is (where x!Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history
X n x n − px (1p) nx VAR(X) = np(1p) = 3* 03 * 07 = 063 SD(X) = np(1p) Calculations shown for Binomial (n=3, p=03) = 0794 Note this is equivalent to counting success = 1 and failure = 0Click here👆to get an answer to your question ️ If p(x) = x^2 2 √(2)x 1 , then p(2√(2)) is equal to Join / Login > 10th > Maths > Polynomials > Relationship between ZeroesNew questions in Mathematics




If It Was X 2 2x 1 X 2 Then How Would You Do It I Let P X 2x2 X Maths Polynomials Meritnation Com




Polynomial 1 Class 9 Notes
p (x)=x²2√2x1 p (2√2)= (2√2)² (2√2*2√2)1 p (2√2)= (2√2)² (2√2)²1 =01 =1 Therefore, the answer is 1 Thank you Hope this will help you mitgliedd1 and 1355 more users found this answer helpfulFinally, using distributive property and foil, you would get x^2xx^23x2=(x^2x2)2x^22x2=x^2x23x^23x=0 3x(x1)x=0 and x=1 ( thats only if the big X is the same as the small xs) You are right, thank you SO MUCH!!Get stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!
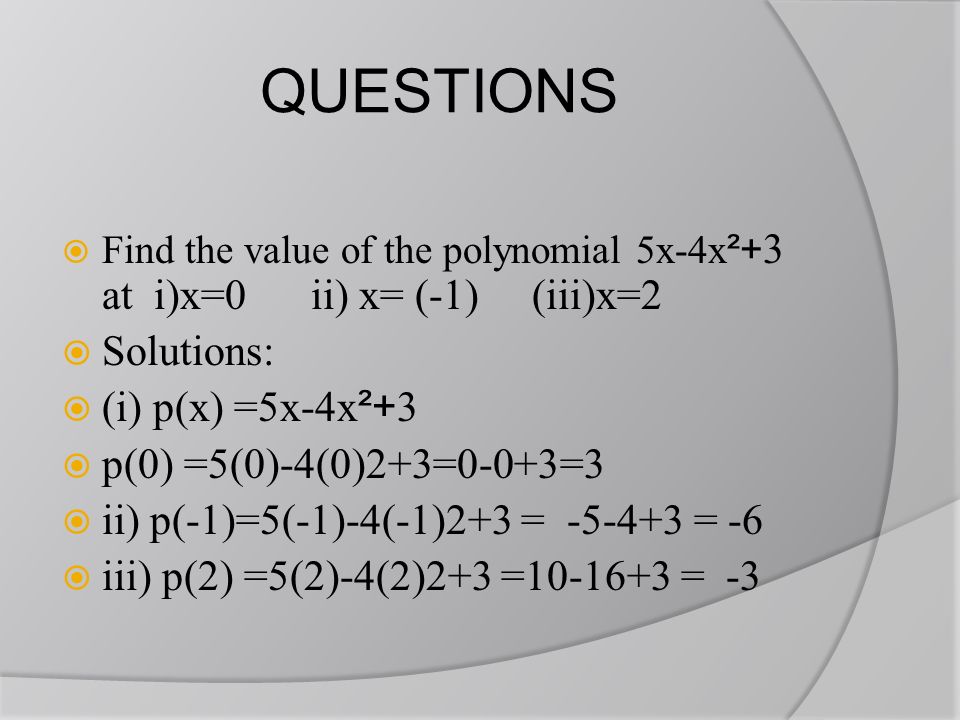



Polynomials Ppt Video Online Download



Find The Values Of P And Q For Which F X 1 Sin 3 X 3cos 2 X If X Pi 2 And P If X Pi 2 Sarthaks Econnect Largest Online Education Community
BASIC STATISTICS 5 VarX= σ2 X = EX 2 − (EX)2 = EX2 − µ2 X (22) ⇒ EX2 = σ2 X − µ 2 X 24 Unbiased Statistics We say that a statistic T(X)is an unbiased statistic for the parameter θ of theunderlying probabilitydistributionifET(X)=θGiventhisdefinition,X¯ isanunbiasedstatistic for µ,and S2 is an unbiased statisticfor σ2 in a random sample 3Westbrass R x 11/2 in PTrap with High Box Flange, Matte Black Reviews, So you can check out the other reviews at AmazoncomThe answer should be 22 As P (Q (x)) = P (x)R (x) P (x) = (x1) (x2) (x3) (Q (x) 1) (Q (x) 2) (Q (x) 3) = (x1) (x2) (x3)R (x) And R (x) is a 3 degree polynomial So, Q (x) should be a 2 degree polynomial We can write R (x) as (xa) (xb) (xb) because it is a 3 degree polynomial
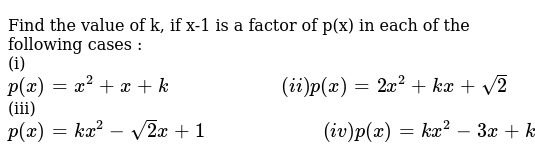



Find The Value Of K If X 1 Is A Factor Of P X In Each Of The Fol




If P X 2x 2 3x 5 Then Find The Value Of P 0 P 1 Whole Devided By P 1 Maths Polynomials Meritnation Com
77k views asked in Class IX Maths by ashu Premium (930 points) If p (x) = x2 – 2√2x 1, then p (2√2) is equal to (a) 0 (b) 1 (c) 4√2 (d) 8 √2 1 polynomials The Attempt at a Solution P (X 1 < X 2 < X 3) = triple integral of e (x1x2x3) dx 2 dx 1 dx 3 as x 2 goes from x 1 to x 3, x 1 goes from 0 to x 2 and x 3 goes from x 2 to infinity When I solve this integral I get 0 for an answer The back of the book suggests the answer is 1/6 Explanation (x −1)(x 2) aaaaaaaaaaaaax − 1 aaaaaaaaaaa × x 2 −−−− − aaaaaaaaaaaaax2 − x aaaaaaaaaaaaaaaa2x − 2 aaaaaaaaaaaaaa¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯x2 x − 2




P 1 Actuary Exam Question Mathematics Stack Exchange



Madasmaths Com Archive Maths Booklets Basic Topics Various Polynomials Exam Questions Intro Pdf
Show 1 and 1 are only solutions to x^2 = 1 (mod p^2) for odd prime p Let p be an odd prime and n be an integer Show that 1 and 1 and the only solutions to x 2 ≡ 1 m o d p n Hint What does a ≡ b m o d m mean, then think a bit for k an integer1x2at Sportwetten und Casino Today`s offer Complete offer Back Sport SportThis preview shows page 123 126 out of 360 pages X 0 P (X 0) A 0 1 B 0 2 C 0 3 D 0 4 (a) 2 pts Fill in the table below with the distribution of Waldo's location at time t = 1 X t P (X 1) A 0 1 * 0 8 0 2 * 0 3 = 0 14 B 0 2 * 0 5 0 1 * 0 2 0 3 * 0 3 = 0 21 C 0 3 * 0 5 0 4 * 0 3 0 2 * 0 2 = 0 31 D 0 4 * 0 7 0 3 * 0 2 = 0 34 (b) 3 pts F T (X) is




Factoring Polynomials Ppt Download




If X 1 2 Is The Zero Of The Polynomial P X 8x3 Ax2 X 2 Find The Value Of A Maths Meritnation Com



0 件のコメント:
コメントを投稿